• Maths Class 10th Constructions

  Chapters

10th Class - Mathematics

  Share

10th Class - Mathematics
  • Text Book Solutions
  • Important Question
  • Question Papers / Notes Download

Solutions

NCERT Solutions for Class 10 Maths Chapter 11 Constructions

NCERT Solutions Exercise 11.1 Class 10 Constructions

In each of the following, give the justification of the construction also:
Question 1.
Draw a line segment of length 7.6 cm and divide it in the ratio 5:8. Measure the two parts.
 

Solution:
Steps of Construction:

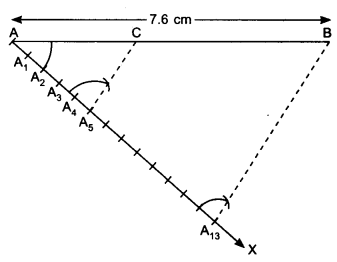
  • 1. Draw a line segment AB = 7.6 cm.
  • 2. Draw an acute angle BAX on base AB. Mark the ray as AX.
  • 3. Locate 13 points A1, A2, A3, …… , A13 on the ray AX so that AA1 = A1A2 = ……… = A12A13
  • 4. Join A13 with B and at A5 draw a line ∥ to BA13, i.e. A5C. The line intersects AB at C.
  • 5. On measure AC = 2.9 cm and BC = 4.7 cm.

Justification:
In ∆AA5C and ∆AA13B,
 

∴ AC : BC = 5 : 8

Question 2.
Construct a triangle of sides 4 cm, 5 cm and 6 cm and then a triangle similar to it whose sides are 2/3 of the corresponding sides of the first triangle.
 

Solution:
Steps of Construction:

  • 1. Construct a ∆ABC with AB = 4 cm, BC = 6 cm and AC = 5 cm.
  • 2. Draw an acute angle CBX on the base BC at point B. Mark the ray as BX.
  • 3. Mark the ray BX with B1, B2, B3 such that
  • BB1 = B1B2 = B2B3
  • 4. Join B3 to C.
  • 5. Draw B2C’ ∥ B3C, where C’ is a point on BC.
  • 6. Draw C’A’ ∥ AC, where A’ is a point on BA.
  • 7. ∆A’BC’ is the required triangle.

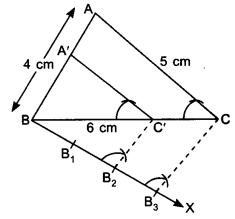
Justification: In ∆A’BC and ∆ABC,

Question 3.
Construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are 7/5 of the corresponding sides of the first triangle.
 

Solution:
Steps of Construction:

  • 1. Draw a ∆ABC with AB = 5 cm, BC = 7 cm and AC = 6 cm.
  • 2. Draw an acute angle CBX below BC at point B.
  • 3. Mark the ray BX as B1, B2, B3, B4, B5, B6 and B7 such that BB1= B1B2 = B2B3 = B3B4 = B4B5 = B5B6 = B6B7.
  • 4. Join B5 to C.
  • 5. Draw B7C’ parallel to B5C, where C’ is a point on extended line BC.
  • 6. Draw A’C’ ∥ AC, where A’ is a point on extended line BA.
  • A’BC’ is the required triangle.

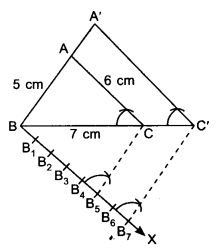
Justification: In ∆ABC and ∆A’BC’,

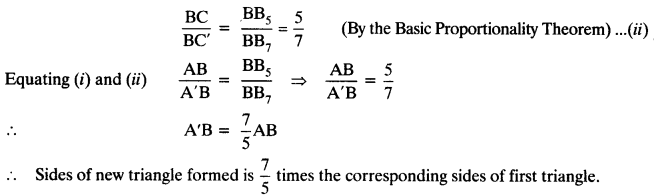
Question 4.
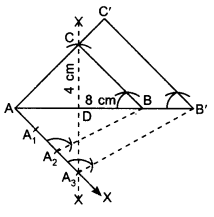
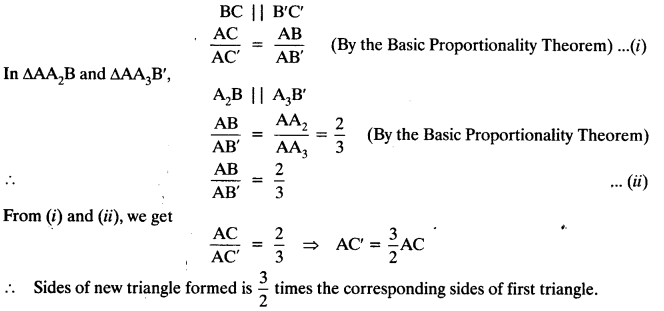
Construct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose sides are 1 1/2 times the corresponding sides of the isosceles triangle.
 

Solution:
Steps of Construction:

  • 1. Draw base AB = 8 cm.
  • 2. Draw perpendicular bisector of AB. Mark CD = 4 cm, on ⊥ bisector where D is mid-point on AB.
  • 3. Draw an acute angle BAX, below AB at point A.
  • 4. Mark the ray AX with A1, A2, A3 such that AA1 =A1A2 = A2A3
  • 5. Join A2 to B. Draw A3B’ ∥ A2 B, where B’ is a point on extended line AB.
  • 6. At B’, draw B’C’ 11 BC, where C’ is a point on extended line AC.
  • 7. ∆AB’C’ is the required triangle.

Justification: In ∆ABC and ∆A’BC’,
 

Question 5.
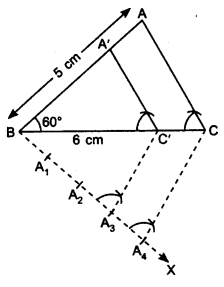
Draw a triangle ABC with side BC = 6 cm, AB = 5 cm and ∠ABC = 60°. Then construct a triangle whose sides are 3/4 of the corresponding sides of the triangle ABC.
 

Solution:
Steps of Construction:

  • 1. Draw a line segment BC = 6 cm and at point B draw an ∠ABC = 60°.
  • 2. Cut AB = 5 cm. Join AC. We obtain a ∆ABC.
  • 3. Draw a ray BX making an acute angle with BC on the side opposite to the vertex A.
  • 4. Locate 4 points A1, A2, A3 and A4 on the ray BX so that BA1 = A1A2 = A2A3 = A3A4.
  • 5. Join A4 to C.
  • 6. At A3, draw A3C’ ∥ A4C, where C’ is a point on the line segment BC.
  • 7. At C’, draw C’A’ ∥ CA, where A’ is a point on the line segment BA.

∴ ∆A’BC’ is the required triangle.

Question 6.
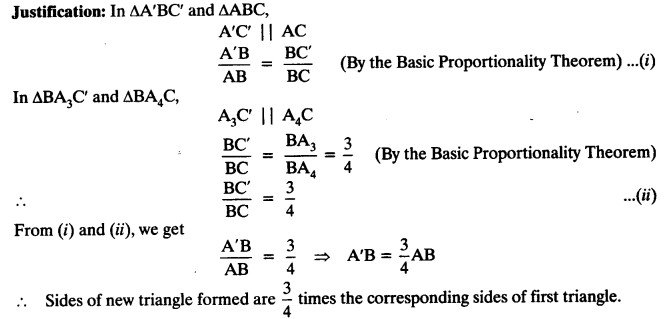
Draw a triangle ABC with side BC = 7 cm, ∠B = 45°, ∠A = 105°. Then, construct a triangle whose sides are 43 times the corresponding sides of ∆ABC.
 

Solution:
In ∆ABC, ∠A + ∠B + ∠C = 180°
⇒ 105° + 45° + ∠C = 180°
⇒ 150° + ∠C = 180°
⇒ ∠C = 30°
Steps of Construction:
1. Draw a line segment BC = 7 cm. At point B, draw an ∠B = 45° and at point C, draw an ∠C = 30° and get ΔABC.
2. Draw an acute ∠CBX on the base BC at point B. Mark the ray BX with B1, B2, B3, B4, such that BB1 = B1B2 = B2B3 = B3B4
3. Join B3 to C.
4. Draw B4C’ ∥ B3C, where C’ is point on extended line segment BC.
5. At C’, draw C’A’ ∥ AC, where A’ is a point on extended line segment BA.
6. ∆A’BC’ is the required triangle.

Justification: In ∆ABC and ∆A’BC’,

Question 7.
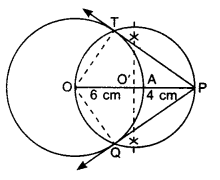
Draw a right triangle in which the sides (other than hypotenuse) are of lengths 4 cm and 3 cm. Then construct another triangle whose sides are 5/3F times the corresponding sides of the given triangle.
 

Solution:
Steps of Construction:

  • 1. Draw a right angled ∆ABC with AB = 4 cm, AC = 3 cm and ∠A = 90°.
  • 2. Make an acute angle BAX on the base AB at point A.
  • 3. Mark the ray AX with A1, A2, A3, A4, A5 such that AA1 = A1A2 = A2A3 = A3A4 = A4A5.
  • 4. Join A3B. At A5, draw A5B’ ∥ A3B, where B’ is a point on extended line segment AB.
  • 5. At B’, draw B’C’ ∥ BC, where C’ is a point on extended line segment AC.
  • 6. ∆AB’C’ is the required triangle.

Justification:
In ∆ABC and ∆AB’C’,

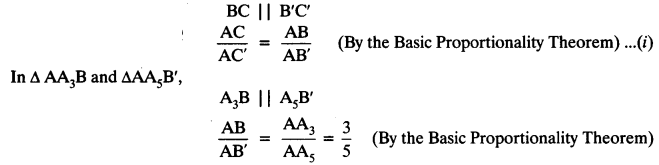
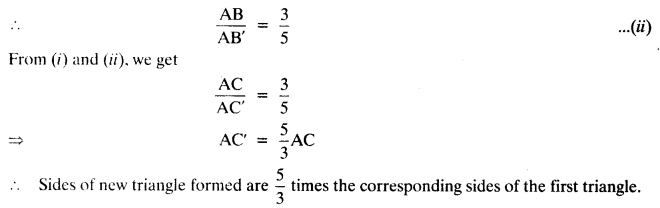
NCERT Solutions Exercise 11.2 Class 10 Constructions

In each of the following, give the justification of the construction also:
Question 1.
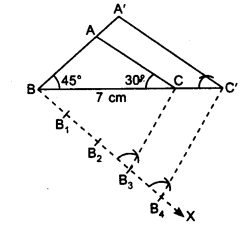
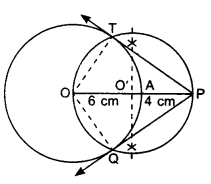
Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
 

Solution:
Steps of Construction:

  • 1. Draw a circle of radius 6 cm.
  • 2. Mark a point P at a distance 10 cm from the centre O.
  • 3. Here OP = 10 cm, draw perpendicular bisector of OP, which intersects OP at O’.
  • 4. Take O’ as centre, draw a circle of radius O’O, which passes through O and P and intersects the previous circle at points T and Q.
  • 5. Join PT and PQ, measure them, these are the required tangents.

Justification:
Join OT and OQ.

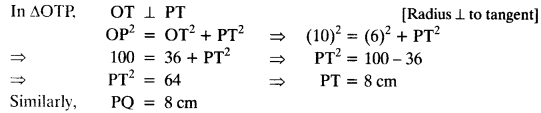
A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ PT = PQ.

Question 2.
Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.
 

Solution:
Steps of Construction:

  • 1. Draw concentric circles of radius OA = 4 cm and OP = 6 cm having same centre O.
  • 2. Mark these circles as C and C’.
  • 3. Points O, A and P lie on the same line.
  • 4. Draw perpendicular bisector of OP, which intersects OP at O’.
  • 5. Take O’ as centre, draw a circle of radius OO’ which intersects the circle C at points T and Q.
  • 6. Join PT and PQ, these are the required tangents.
  • 7. Length of these tangents are approx. 4.5 cm.

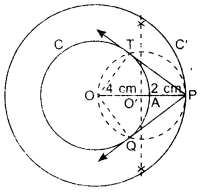
Justification:
Join OT and OQ.

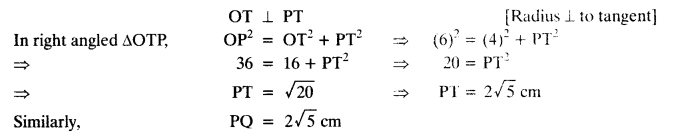
A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ PT = PQ.

Question 3.
Draw a circle of radius 3 cm. Take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre. Draw tangents to the circle from these two points P and Q.
 

Solution:
Steps of Construction:

 

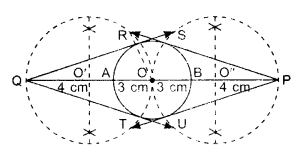
  • 1. Draw a circle of radius 3 cm, having centre O. Mark this circle as C.
  • 2. Mark points P and Q along its extended diameter such that OP = 7 cm and OQ = 7 cm.
  • 3. Draw perpendicular bisector of OQ, intersecting OQ at O’.
  • 4. Draw perpendicular bisector of OP intersecting OP at O”.
  • 5. Take O’ as centre and draw a circle of radius OO’ which passes through points O and Q, intersecting circle C at points R and T.
  • 6. Take O” as centre and draw a circle of radius O”P which passes through points O and P, intersecting the, circle C at points S and U.
  • 7. Join QR and QT; PS and PU, these are the required tangents.
     

Justification:
Join OR.
In ∆OQR,
OR ⊥ QR [Radius ± to tangent]
OQ2 = OR2 + QR2
⇒ (7)2 = (3)2 + QR2 ⇒ 49 = 9 + OR2
⇒ 40 = QR2 ⇒ QR = 2 √10 cm
Similary,
QT = 2 √10 cm
PS = 2 √10 cm
PU = 2 √10 cm
A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ QR = QT and PS = PU

Question 4.
Draw a pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 60°.
 

Solution:
Steps of Construction:

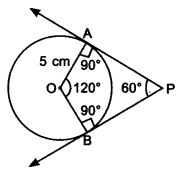
  • 1. Draw a circle of radius 5 cm.
  • 2. As tangents are inclined to each other at an angle of 60°.
  • ∴ Angle between the radii of circle is 120°. (Use quadrilateral property)
  • 3. Draw radii OA and OB inclined to each other at an angle 120°.
  • 4. At points A and B, draw 90° angles. The arms of these angles intersect at point P.
  • 5. PA and PB are the required tangents.
     

Justification:
In quadrilateral AOBP,
AP and BP are the tangents to the circle.
Join OP.
In right angled AOAP, OA ⊥ PA [Radius is ⊥ to tangent]

A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ PA = PB

Question 5.
Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
 

Solution:
Steps of Construction:

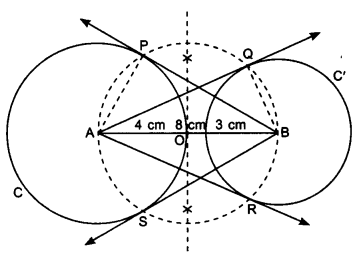
  • 1. Draw a line segment AB = 8 cm.
  • 2. Taking A as centre draw a circle C of radius 4 cm and taking B as centre draw a circle C’ of radius 3 cm.
  • 3. Draw perpendicular bisector of AB, which intersects AB at point O.
  • 4. Taking point O as centre draw a circle of radius 4 cm passing through points A and B which intersect circle C at P and S and circle C’ at points R and Q.
  • 5. Join AQ, AR, BP and BS. These are the required tangents.
     

Justification:
Join AP.

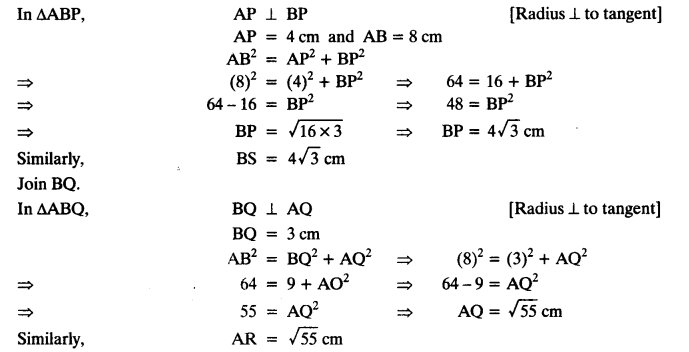
A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ AQ = AR and BP = BS.

Question 6.
Let ABC be a right triangle in which AB = 6 cm, BC = 8 cm and ZB = 90°. BD is the perpendicular Burn B on AC. The circle through B, C, D is drawn. Construct the tangents from A to this circle.
 

Solution:
Steps of Construction:

  • 1. Draw a right triangle ABC with AB = 6 cm, BC = 8 cm and ZB = 90°.
  • 2. From B, draw BD perpendicular to AC.
  • 3. Draw perpendicular bisector of BC which intersect BC at point O’.
  • 4. Take O’ as centre and O’B as radius, draw a circle C’ passes through points B, C and D.

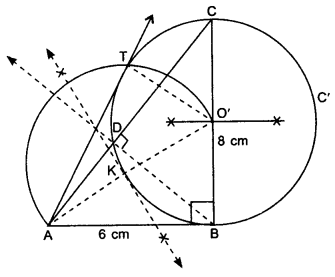
5. Join O’A and draw perpendicular bisector of O’A which intersect O’A at point K.
6. Take K as centre, draw an arc of radius KO’ intersect the previous circle C’ at T.
7. Join AT, AT is required tangent.
Justification:
∠BDC = 90°
∴ BC acts as diameter.
AB is tangent to circle having centre O’

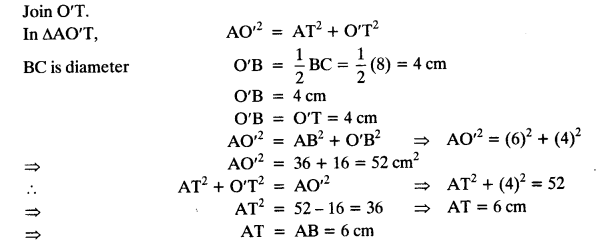
A pair of tangents can be drawn to a circle from an external point outside the circle. These two tangents are equal in lengths.
∴ AB = AT.

Question 7.
Draw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circle.
 

Solution:
Steps of Construction:
1. Draw a circle C’ with the help of a bangle, for finding the centre, take three non-collinear points A, B and C, lying on the circle. Join AB and BC and draw perpendicular bisector of AB and BC, both intersect at a point O,
‘O’ is centre of the circle.

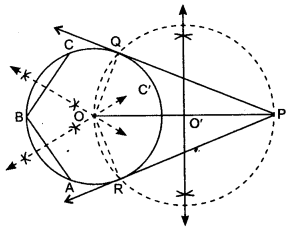
2. Take a point P outside the circle. Join OP.
3. Draw perpendicular bisector of OP, which intersects OP at point O’.
4. Take O’ as the centre with OO’ as radius draw a circle which passes through O and P, intersecting previous circle C’ at points R and Q.
5. Join PQ and PR.
6. PQ and PR are the required pair of tangents.
Justification:
Join OQ and OR.
In AOQP and AOPR, OQ = OR [Radii of the circle]
OP = OP [Common]
∠Q = ∠R = 90° [Radius is ⊥ to tangent]

∆OQP ≅ ∆ORP [by RHS]
∴ PQ = PR [By C.P.C.T]
A pair of tangents can be drawn to a circle from an external point lying outside the circle. These two tangents are equal in lengths.
∴ PQ = PR

Important Question

NCERT CBSE for Class 10 Maths Chapter 11 Constructions Important Questions

Constructions Class 10 Important Questions Short Answer-I (2 Marks)

Question 1.
Draw a line segment of length 6 cm. Using compasses and ruler, find a point P on it which divides it in the ratio 3 : 4.
Year of Question: (2011D)

Solution:
Hence, PA : PB = 3 : 4

Question 2.
Draw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such that AP/AB=3/5.
Year of Question: (2011OD)

Solution:
AB = 7 cm, AB = AP/AB=3/5 . [Given
∴ AP : PB = 3 : 2
Hence, AP : AB = 3 : 5 or AP/AB=3/5

Constructions Class 10 Important Questions Short Answer-II (3 Marks)

Question 3.
Draw a triangle ABC in which AB = 5 cm, BC = 6 cm and ∠ABC = 60°. Then construct a triangle whose sides are 5/7 times the corresponding sides of ∆ABC.
Year of Question: (2011D)
Solution:
In ∆ABC
AB = 5 cm
BC = 6 cm
∠ABC = 60°
Hence, ∆A’BC’ is the required ∆.
Question 4.
Construct a triangle with sides 5 cm, 5.5 cm and 6.5 cm. Now construct another triangle, whose sides are 35 times the corresponding sides of the given triangle.
Year of Question: (20140D)

Solution:
∴ ∆A’BC’ is the required ∆.

Question 5.
Construct a right triangle in which the sides, (other than the hypotenuse) are of length 6 cm and 8 cm. Then construct another triangle, whose sides are 3/5 times the corresponding sides of the given triangle.
Year of Question: (2012D)
Solution:
Here AB = 8 cm, BC = 6 cm and
Ratio = 3/5 of corresponding sides
∴ ∆A’BC’ is the required triangle.
Question 6.
Draw a triangle PQR such that PQ = 5 cm, ∠P = 120° and PR = 6 cm. Construct another triangle whose sides are 3/4 times the corresponding sides of ∆PQR.
Year of Question: (2011D)

Solution:
In ∆PQR,
PQ = 5 cm, PR = 6 cm, ∠P = 120°
∴ ∆P’OR’ is the required ∆.

Question 7.
Draw a triangle ABC with BC = 7 cm, ∠B = 45° and ∠C = 60°. Then construct another triangle, whose sides are 3/5 times the corresponding sides of ∆ABC.
Year of Question: (2012OD)
Solution:
Here, BC = 7 cm, ∠B = 45°, ∠C = 60° and ratio is 35 times of corresponding sides
∴ ∆A’BC’ is the required triangle.
Question 8.
Construct a triangle with sides 5 cm, 4 cm and 6 cm. Then construct another triangle whose sides are 2/3 times the corresponding sides of first triangle.
Year of Question: (2013D)

Solution:

Steps of Construction:
Draw ∆ABC with AC = 6 cm, AB = 5 cm, BC = 4 cm.
Draw ray AX making an acute angle with AÇ.
Locate 3 equal points A1, A2, A3 on AX.
Join CA3.
Join A2C’ ‖ CA3.
From point C’ draw B’C’ ‖ BC
. ∴ ∆A’BC’ is the required triangle.

Question 9.
Draw a pair of tangents to a circle of radius 3 cm, which are inclined to each other at an angle of 60°.
Year of Question:(2011OD)

Solution:
∴ PA & PB are the required tangents.

Question 10.
Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm.
Year of Question:(2013D)

Solution:
Steps of Construction:
Draw two circles with radius OA = 4 cm and OP = 6 cm with O as centre. Draw ⊥ bisector of OP at M. Taking M as centre and OM as radius draw another circle intersecting the smaller circle at A and B and touching the bigger circle at P. Join PA and PB. PA and PB are the required tangents.
Verification:
In rt. ∆OAP,
OA2 + AP2= OP2 . [Pythagoras’ theorem
(4)2 + (AP)2 = (6)2
AP2 = 36 - 16 = 20
AP = + √20=√4×5 = 2√5 = 2(2.236) = 4.472 = 4.5 cm By measurement, ∴ PA = PB = 4.5 cm

Question 11.
Draw a pair of tangents to a circle of radius 4.5 cm, which are inclined to each other at an angle of 45°.
Year of Question:(2013OD)

Solution:
Draw ∠AOB = 135°, ∠OAP = 90°, ∠OBP = 90°
∴ PA and PB are the required tangents.

Question 12.
Draw two tangents to a circle of radius 3.5 cm, from a point P at a distance of 6.2 cm from its cehtre.
Year of Question:(2013OD)

Solution:
OP = OC + CP = 3.5 + 2.7 = 6.2 cm
Hence AP & PB are the required tangents.

Question 13.
Draw a right triangle ABC in which AB = 6 cm, BC = 8 cm and ∠B = 90°. Draw BD perpendicular from B on AC and draw a circle passing through the points B, C and D. Construct tangents from A to this circle.
Year of Question:(2014D, 2015OD)

Solution:
Steps of Construction:
Draw BC = 8 cm.
From B draw an angle of 90°.
Draw an arc BA = 6 cm cutting the angle at A.
Join AC. ∴ ∆ABC is the required ∆.
Draw ⊥ bisector of BC cutting BC at M.
Take Mas centre and BM as radius, draw a circle.
Take A as centre and AB as radius draw an arc cutting the circle at E. Join AE.
AB and AE are the required tangents.
Justification: ∠ABC = 90° .[Given
Since, OB is a radius of the circle.
∴ AB is a tangent to the circle.
Also, AE is a tangent to the circle.

Question 14.
Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
Year of Question: (2014OD)

Solution:
Draw two circles on A and B as asked.
Z is the mid-point of AB.
From Z, draw a circle taking ZA = ZB as radius,
so that the circle intersects the bigger circle at M and N and smaller circle at X and Y.
Join AX and AY, BM and BN.
BM, BN are the reqd. tangents from external point B.
AX, AY are the reqd. tangents from external point A.
Justification:
∠AMB = 90° .[Angle in a semi-circle
Since, AM is a radius of the given circle.
∴ BM is a tangent to the circle
Similarly, BN, AX and AY are also tangents.

Constructions Class 10 Important Questions Long Answer (4 Marks).

Question 15.
Draw a triangle ABC with side BC = 7 cm, ∠B = 45° and ∆A = 105°. Then construct a triangle whose sides are 35times the corresponding sides of ∆ABC.
Year of Question:(2011D)

Solution:
In ∆ABC, ∠A + ∠B + ∠C = 180° . [angle sum property of a ∆
105° + 45° + C = 180°
∠C = 180° - 105° - 45o = 30°
BC = 7 cm
∴ ∆A’BC’ is the required ∆.

Question 16.
Draw a triangle ABC with side BC = 6 cm, ∠C = 30° and ∠A = 105°. Then construct another triangle whose sides are 2/3 times the corresponding sides of ∆ABC.
Year of Question:(2012D)

Solution:
Here, BC = 6 cm, ∠A = 105° and ∠C = 30°
In ∆ABC,
∠A + ∠B + ∠C = 180° .[Angle-sum-property of a ∆
105° + ∠B + 30° = 180°
∠B = 180° - 105° - 30° = 45°
∴ ∆A’BC’ is the required ∆.

Question 17.
Draw a triangle with sides 5 cm, 6 cm and 7 cm. Then construct another triangle whose sides are 2/3 times the corresponding sides of the first triangle.
Year of Question:(2012OD)

Solution:
Here, AB = 5 cm, BC = 7 cm, AC = 6 cm and ratio is 2/3 times of corresponding sides.
∴ ∆A’BC’ is the required triangle.

Question 18.
Construct an isosceles triangle whose base is 6 cm and altitude 4 cm. Then construct another triangle whose sides are 3/4 times the corresponding sides of the isosceles triangle.
Year of Question:(2015D)
Solution:
∴ ∆A’BC’ is the required triangle.
Question 19.
Draw a line segment AB of length 7 cm. Taking A as centre, draw a circle of radius 3 cm and taking B as centre, draw another circle of radius 2 cm. Construct tangents to each circle from the centre of the other circle.
Year of Question:(2015D)

Solution:
Draw two circles on A and B as asked.
Z is the mid-point of AB.
From Z, draw a circle taking ZA = ZB as radius,
so that the circle intersects the bigger circle at M and N and smaller circle at X and Y.
Join AX and AY, BM and BN.
BM, BN are the required tangents from external point B.
AX, AY are the required tangents from external point A.
Justification:
∠AMB = 90° .[Angle in a semi-circle
Since, AM is a radius of the given circle.
∴BM is a tangent to the circle
Similarly, BN, AX and AY are also tangents.

Question 20.
Construct a ∆ABC in which AB = 6 cm, ∠A = 30° and ∆B = 60°. Construct another ∆AB’C’ similar to ∆ABC with base AB’ = 8 cm.
Year of Question:(2015OD)

Solution:
Steps of construction:
Draw a ∆ABC with side AB = 6 cm, ∠A = 30° and ∠B = 60°.
Draw a ray AX making an acute angle with AB on the opposite side of point C.
Locate points A1, A2, A3 and A4 on AX.
Join A3B. Draw a line through A4 parallel to A3B intersecting extended AB at B’.
Draw a line parallel to BC intersecting ray AY at C’.
Hence, ∆AB’C’ is the required triangle.

Question 21.
Construct a triangle ABC in which AB = 5 cm, BC = 6 cm and ∠ABC = 60°. Now construct another triangle whose sides are 5/7 times the corresponding sides of ∆ABC.
Year of Question:(2015OD)

Solution:
In ∆ABC, AB = 5 cm; BC = 6 cm; ∠ABC = 60°
∴ ∆A’BC’ is the required ∆.

Question 22.
Construct a triangle ABC in which BC = 6 cm, AB = 5 cm and ∠ABC = 60°. Then construct another triangle whose sides are 3/4 times the corresponding sides of ∆ABC.
Year of Question:(2016D)

Solution:
Steps of Construction:
Draw ∆ABC with the given data.
Draw a ray BX downwards making an acute angle with BC.
Locate 4 points B1, B2, B3, B4, on BX, such that BB1 = B1B2 = B2B3 = B3B4.
Join CB4.
From B3 draw a line C’B3 ‖ CB4 intersecting BC at C’.
From C’ draw A’C’ ‖ AC intersecting AB at B’.
Then ∆A’BC’ is the required triangle.
Justification:

Question 23.
Draw a triangle ABC with BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are 4/5 times the corresponding sides of ∆ABC.
Year of Question:(2016D)

Solution:
In ∆ABC, ∠A + ∠B + ∠C = 180° ..[Angle-sum-property of a ∆
105° + 45° + ∠C = 180°
∠C = 180° - 105° - 45° = 30°
∴ ∆A’BC’ is the required triangle.

Question 24.
Draw an isosceles ∆ABC in which BC = 5.5 cm and altitude AL = 3 cm. Then construct another triangle whose sides are 3/4 of the corresponding sides of ∆ABC.
Year of Question:(2016OD)

Solution:
∴ ∆A’BC’ is the required ∆.

Question 25.
Draw a triangle with sides 5 cm, 6 cm and 7 cm. Then draw another triangle whose sides are 4/5 of the corresponding sides of first triangle.
Year of Question:(2016OD)

Solution:
∴ ∆A’BC’ is the required ∆.

Question 26.
Draw two concentric cirlces of radii 3 cm and 5 cm. Construct a tangent to smaller circle from a point on the larger circle. Also measure its length.
Year of Question:(2016D)

Solution:
We have, OD = 3 cm and OP = 5 cm
PA and PB are the required tangents
By measurement PA = PB = 4 cm.

Question 27.
Draw a circle of radius 4 cm. Draw two tangents to the circle inclined at an angle of 60° to each other.
Year of Question:(2013, 2016OD)

Solution:
Draw a circle with O as centre and radius 4 cm.
Draw any ∠AOB = 120°. From A and B draw ∠PAO = ∠PBO = 90° which meet at P.
∴ PA and PB are the required tangents.

Question Papers / Notes Download

Videos